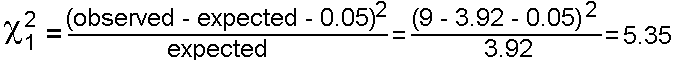

Under the assumption that the observed is a Poisson variable with a mean equal to the expected, and based on the normal approximation to the Poisson distribution, the chi-square with one degree of freedom is
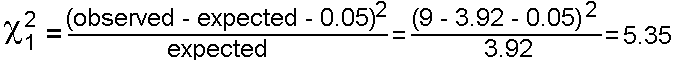
Consulting tables for the chi-square statistic with one degree of freedom, we find that the p-value for 5.35 is approximately 0.02, which means that the probability of finding nine or more observed cancers when only 3.92 were expected, if the null hypothesis of no exposure effect were true, was only 2%. Hence, we can reject the null hypothesis and find that the rate ratio is significantly elevated above the null rate ratio of 1.0. The 95% test-based confidence interval is 2.30(1± 1.96/c), or (1.13, 4.66).
The reader should note that more precise tests of significance and confidence limits are easily available via various software packages. We have used these approximations here because they are easily calculated by hand.
The above calculation treats the expected number of larynx cancers as invariant. Actually, due to the adjustment of the expected for smoking and drinking (15% increase), there is some variation associated with the expected number, which we have not considered here. This would tend to increase the width of the confidence interval for the rate ratio, althougl not dramatically.